Published online Nov 12, 2014. doi: 10.5318/wjo.v4.i4.140
Revised: July 24, 2014
Accepted: September 23, 2014
Published online: November 12, 2014
Processing time: 199 Days and 7.5 Hours
This review summarises the current evidence base and provides guidelines for obtaining good refractive outcomes following cataract surgery. Important background information is also provided. In summary, the requirements are: (1) standardisation of biometry equipment used for axial length and keratometry measurement and the use of optical or immersion ultrasound biometry; (2) sutureless cataract surgery with “in the bag” intraocular lens (IOL) placement; (3) an appropriate 3rd, 4th or 5th Generation IOL power formula should be used; (4) IOL formula constants must be optimized; (5) under certain conditions, the refractive outcome of the 2nd eye can be improved based on the refractive error of the first eye; and (6) results should be audited for refinement and to ensure that standards are met.
Core tip: The requirements for good refractive outcomes in cataract surgery are: (1) standardisation of biometry equipment used for axial length and keratometry measurement and the use of optical or immersion ultrasound biometry; (2) sutureless cataract surgery with “in the bag” intraocular lens placement; (3) an appropriate 3rd, 4th or 5th Generation intraocular lens (IOL) power formula should be used; (4) IOL formula constants must be optimized; (5) under certain conditions, the refractive outcome of the 2nd eye can be improved based on the prediction error of the cataract surgery for the first eye; and (6) results should be audited for refinement and to ensure that standards are met.
- Citation: Aristodemou P, Cartwright NEK, Sparrow JM, Johnston RL. Improving refractive outcomes in cataract surgery: A global perspective. World J Ophthalmol 2014; 4(4): 140-146
- URL: https://www.wjgnet.com/2218-6239/full/v4/i4/140.htm
- DOI: https://dx.doi.org/10.5318/wjo.v4.i4.140
Advances in technology and improvements in technique have made cataract surgery safe and effective at restoring vision to millions of patients worldwide. Phacoemulsification with intracapsular intraocular lens (IOL) implantation has been universally adopted in developed countries and manual small incision cataract surgery has revolutionized service delivery elsewhere. Achieving the desired refractive outcome is now a primary aim of surgery and so can be used as a measure of the quality of clinical services. In developed countries, patients expect reduced spectacle dependence and in developing countries residual uncorrected high refractive error remains an important cause of visual disability, which can be caused by inappropriate selection of IOL power at surgery[1]. This article reviews the evidence base and offer guidelines to achieve optimal refractive outcomes.
Conventional extracapsular cataract surgery with a large corneal section requiring sutures has declined in popularity. Its main disadvantages were the delay in visual rehabilitation due to the induction of corneal astigmatism as well as the need to remove corneal sutures following surgery[2]. Furthermore, sulcus IOL placement, makes the actual post-operative IOL position, and hence refraction, less predictable[3]. Continuous curvilinear capsulorhexis allows for predictable placement of the IOL in the capsular bag and small self sealing corneal incisions induce less post-operative astigmatism[4-6]. Manual small incision cataract surgery can also result in low amount of induced astigmatism, especially when a temporal approach is used[7].
Optimal refractive outcomes can be achieved both in phacoemulsification and small incision manual cataract extraction when the following conditions are met: (1) reproducible measurement of axial length and keratometry (preferably but not necessarily with optical biometry), (2) good surgical technique with a low rate of posterior capsular rupture; (3) in-the-bag IOL placement and a capsulorhexis size smaller than the optic diameter; (4) appropriate choice of IOL power formula and, most importantly, (5) availability of post operative refraction; and (6) adjustment of the IOL formula constant (optimization) for the specific IOL model and the specific methods of biometry used.
The measurement of the physical dimensions of the eye is necessary to calculate the intraocular lens power. The minimum measurements required are axial length and corneal power. Many suitable devices can take these measurements but all these have systematic differences in measurement. It is therefore very important that the intraocular lens formula is used with the correct formula constant that corrects for these systematic differences in measurement[4]. This not only applies for the axial length measurement method but also for the keratometry method.
Axial Length measurement can be performed using ultrasound or optical methods. Optical methods use partial coherence interferometry or low coherence reflectometry[8]. Their advantages over ultrasound methods are that they are non-contact, precise and reproducible and have a low dependence on operator skill. Optical methods are considered to be the standard of care in developed countries. Nevertheless, they are more expensive and less portable than ultrasound methods and cannot provide measurements in the presence of dense axial lens opacities. Contact ultrasound methods give systematic errors in measurement of the axial length due to the inadvertent compression of the cornea[9] and the magnitude of error is influenced by the operator experience[10]. Immersion ultrasound techniques do not cause corneal compression and can deliver refractive outcomes similar to optical methods[11].
Modern optical biometry devices have in-built keratometers, which enable very good refractive outcomes with respect to correcting spherical error. It is outside the scope of this review to discuss the assessment and correction of astigmatism at the same time as performing cataract surgery. Published optical IOL formula constants for each IOL model are derived from axial length and keratometric data obtained from each device. These published IOL constants can be used with confidence by surgeons when they start using a new IOL model, provided they are using the same instrument.
Combining separate axial length and keratometry instruments (when for example ultrasound is used for axial length measurement) can also give very good results but departments must make sure that they optimize their IOL formula constants based on their data for the specific set of instruments used. Manufacturers’ IOL constants can not be relied upon for good refractive outcomes.
Newer biometry formulae use one or more of the following biometric measurements: Anterior Chamber Depth, Lens thickness, White to White diameter, age and preoperative refraction. The benefits of using these additional measurements are not proven and excellent results can be obtained using a combination of 3rd generation IOL formulae with optimized IOL constants.
For established eye departments where the supply of electricity is reliable, optical biometry has clear advantages over ultrasound biometry as very precise measurements can be obtained quickly and without any contact of any instrument with the eye. In the setting of limited resources, ultrasound axial length measurements can offer good outcomes, especially when an immersion technique is used. The added time required for traditional immersion techniques with a shell have been a limiting factor with respect to their adoption as it would affect the flow of patients in high volume practices. Newer immersion techniques include the use of a clip-on attachment that requires minimal amount of saline or the use of lubricant eye gel as a coupling agent. These immersion techniques are more time efficient than using a traditional immersion shell and offer advantages in precision over contact methods. In cases where electricity supply is not reliable, both ultrasound biometry and keratometry are available as portable battery operated units. Some portable keratometers are combined with an autorefractor, which is very valuable as IOL constant optimization depends on the availability of post-operative refraction data. The international agency for the prevention of blindness publishes a standard list of equipment for Vision 2020 eye care service units and this can be used for guidance[12,13].
The evolution of IOL power calculation formulae has consistently improved the predictability of refractive outcomes, since Fyotorov’s first IOL formula. There are now a plethora of IOL formulae but only some of these are still considered fit for purpose. Table 1 summarizes the IOL formulae found in biometry instruments, along with comments for each one.
| Name of formula | Variables required | Comments |
| SRK I and Binkhorst I 1st Generation | Keratometry, Axial Length | Obsolete High levels of error, should not be used |
| SRK II and Binkhorst II 2nd Generation | Keratometry, Axial Length | Obsolete High levels of error, should not be used |
| Holladay 1 3rd Generation | Keratometry, Axial Length | Trend toward better outcomes for eyes between 22.00 mm and 26.00 mm, compared to other 3rd generation[14] |
| SRK/T 3rd Generation | Keratometry, Axial Length | Better outcomes for eyes over 26.00 mm, compared to other 3rd generation formulae[14] |
| Hoffer Q 3rd Generation | Keratometry, Axial Length | Better outcomes for eyes under 22.00 mm, compared to other 3rd generation[14] |
| T2 3rd Generation | Keratometry, Axial Length | Very good outcomes for eyes over 22.00 mm[15] It corrects the cusp phenomenon, an error observed using the SRK/T on certain eyes[16] |
| Holladay 2 4th Generation | Keratometry, Axial Length, Anterior Chamber Depth, Lens Thickness, Horizontal White to White, Age, Pre operative refraction | No data has been reported showing an advantage over an appropriately selected 3rd generation formula |
| Olsen 4th Generation | Keratometry, Axial Length, Anterior Chamber Depth, Lens Thickness, Horizontal White to White | There is eidence suggestive of improved performance over 3rd generation formulae for eyes with axial length between 20.00 and 26.00 mm[17,18] |
| Haigis 5th Generation | Keratometry, Axial Length, Anterior Chamber Depth | Very good outcomes for eyes across the axial length range and best reported outcomes for eyes longer than 28.00 mm[19] For best results, three IOL constants need to be optimized requiring data from at least 500 eyes |
Implanting a single power IOL without biometry assessment used to be common in developing countries as it was said that certain populations do not have as high variability in axial length as in developed countries[20]. Nevertheless, choosing an appropriate IOL power based on pre-operative biometry promises improved unaided vision[21] and much more predictable refractive outcomes. Many patients in developing countries do not have access to optical correction and therefore high refractive errors are a significant cause of visual disability[22].
For the majority of cataract surgery cases worldwide, the biometric measurements that can be practically assessed are axial length and keratometry. These can be performed at a low cost using portable battery operated instruments. Using axial length and keratometry, the refractive outcomes of cataract surgery have the potential to reach a very high level of accuracy and precision provided that a number of conditions are met.
An appropriate 3rd generation IOL power formula should be used: The Hoffer Q should be used for eyes less than 22.00mm, the Holladay 1 should be used for eyes between 22.00 and 26.00 and the SRK/T is more appropriate for eyes over 26.00 mm. These formulae are available in commercial biometry instruments. The T2 formula has addressed a source of error inherent to the SRK/T formula, called the cusp phenomenon and can therefore be used in eyes 22.00 or longer[15]. Although the T2 formula has been published, it is not currently included in commercial biometry products. The formula is available as an Excel spreadsheet at http://www.richardsheard.net/T2Formula.aspx. Previous generation two-variable formulae are obsolete and should not be used. These include the SRK-I, SRKII, Binkhost I and II, and the original Hoffer formula.
An optimized IOL constant should be used: The IOL constant value depends on (1) the IOL model; (2) the biometry instruments used; and (3) the position of the IOL implantation (in the bag vs sulcus). These factors are far more important than any differences between surgeons, something formerly thought to be important. Indeed for small incision phacoemulsification surgery and optical biometry it has been demonstrated that there are no significant differences between most surgeon’s optimized constants, only between methods of measurement.
It is worth noting that using manufacturer’s (non-optimized) constants even with an appropriate 3rd generation IOL formula can give very poor refractive outcomes, which are usually in the hyperopic direction when using optical biometry[4]. The IOL constant that is contained on the IOL packaging is often not appropriate for IOL calculations. When starting to use a new IOL implant, it is suggested that published IOL constants are used[23]. For ultrasound biometry, the manufacturer may have data to help departments until they have enough cases to optimize their own IOL constants.
If an incorrect IOL constant is used, the formula would give results which are systematically skewed towards the hypermetropic or myopic end and result in consistently poor outcomes. The optimization of the IOL constant “resets” the mean error of the formula to 0. This means that on average the refractive aim and the post op refraction are the same. From there on, the method of biometry and the appropriateness of the IOL formula help minimise the distribution of errors.
The Holladay 2 was the first published formula that used these variables, but its code has never been available in the public domain. Later on, Thomas Olsen and Wolfgang Haigis separately published their own eponymous IOL formulae and these have been published[17,24]. The evidence so far suggests that the Olsen formula has a statistically significant advantage over the Hoffer Q, the Holladay 1 and the SRK/T in eyes between 20.00 and 26.00 mm[17,18]. The Haigis formula with all three IOL constants optimized has been found to perform very well across the axial length range as well as giving a lower prediction error in very myopic eyes, compared to the SRK/T[19]. We could not find any published evidence showing that the Holladay 2 formula performs better than an appropriately selected 3rd generation IOL formula.
The need to describe and quantify refractive outcomes arises from the fact that in many cases, the predicted postoperative refraction is not the same as the actual (or observed) post operative refraction. Observed refraction minus the Predicted Refraction is the Refraction Error. For example if for a patient the refractive aim with the chosen IOL power is -0.53 D and the actual post operative result was -0.25 D, then the Prediction Error for this eye would be +0.28D.
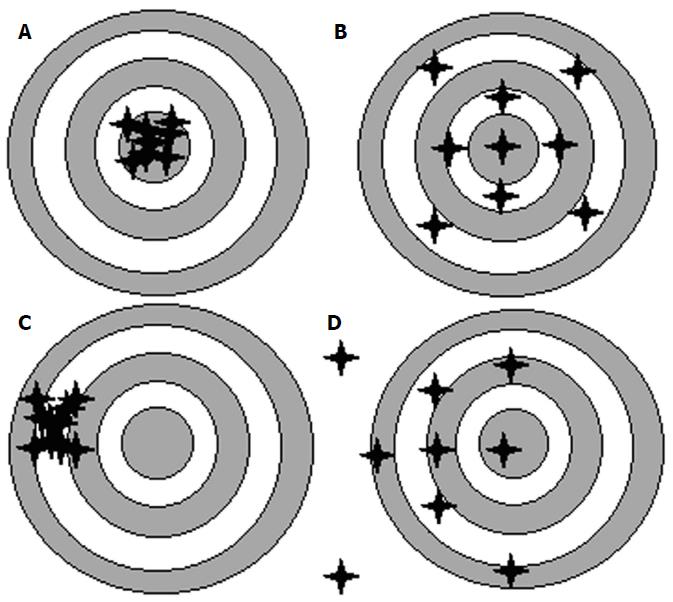
When describing refractive outcomes for a large sample of eyes, the measures need to quantify: (1) how close to zero the average prediction error of this sample of eyes is (Mean Error); and (2) how widely dispersed are the errors spread around this mean. The approach of choosing an appropriate IOL power could be likened to a rifle competition. The success of using a rifle depends not only on the technology of the aiming device it carries but also on whether the aiming device has been appropriately calibrated. Mean Error is a measure of Accuracy, i.e., an AVERAGE measure of how close most shots hit the target. On the other hand, Precision represents the spread of the shots around the mean, and this can be measured by the Standard Deviation of the Prediction Error. Figure 1 gives examples of targets shot with: (1) High Accuracy and High Precision; (2) High Accuracy and Low Precision; (3) Low Accuracy and High Precision; and (4) Low Accuracy and Low Precision.
In the same way, in order to achieve good refractive outcomes in cataract surgery, it is not enough to use appropriate biometry methods and formulae. The calculations need to be calibrated (or optimized) in order to achieve the target refraction for the majority of eyes. The availability of good biometry methods and modern biometry formulae has improved the precision of achieving the refractive target. Nevertheless, this is not enough and surgeons need to use an appropriately Optimized Formula Constant for the particular IOL model used in order to correct the accuracy of the results. There is a very good example of an eye department in the United Kingdom, which made the transition from ultrasound to optical biometry and subsequently audited their refractive outcomes[25]. Their initial results showed that when the manufacturer’s IOL constant was used with optical biometry, patients became on average 0.63D more hypermetropic than expected with only 65% achieving refraction within 1.0 Dioptre from target. Following adjustment of the IOL constant based on audit results, the Mean Error was reduced to -0.14D and 95% of cases achieved refraction within 1.0D of their target. Using the rifle paradigm, the move from ultrasound to optical biometry can be likened to an upgrade of the aiming device of the rifle. The new aiming device was not calibrated and therefore was consistently resulting in shots hitting +0.63D away from target, despite the fact that these shots were tightly grouped. The adjustment of the IOL constant moved the average deviation closer to zero.
Optimizing the IOL constants provides a greater magnitude of improvement in results than choosing the correct IOL formula for a given axial length range[14].
When using a new IOL model, formula constants should be obtained from a reputable source such as the manufacturer or the ULIB website and should be appropriate for the method of measurement used (i.e., for the specific instruments used for axial length and keratometry measurement). The refractive outcomes using this IOL model should be audited and once more than 100 eyes are obtained, the IOL constant may be modified in order to improve future refractive outcomes.
Most IOL constant optimization protocols define a minimum post operative time period from cataract surgery for refractive data collection. This is usually set at 1 month, by which time post operative refraction is considered stable. This should be the standard for most eye units. In situations where patients travel a large distance and they are only available on day 1 post operatively (such as in eye camps), day one autorefraction is a reasonably reliable source of post op refraction, which can be performed by non medical staff and therefore have a small impact on staff resources[21]. In such circumstances cases, day one best corrected visual acuity of 6/18 may be used as the minimum level of VA in order for cases to be included for IOL constant optimization.
For 3rd generation IOL formulae, there should be around 100 such eyes to calculate an optimized IOL constant. The data required are: (1) preoperative Axial length; (2) preoperative keratometry; (3) IOL model and power used; (4) IOL constant used; (5) post operative refraction (spherical equivalent); and (6) post operative visual acuity. Appropriate eyes for inclusion are those that had uncomplicated cataract surgery with in the bag IOL implantation and a final best corrected visual acuity of 6/12 or better (or minimum BCVA 6/18 for day 1 refraction in eye camps). Eyes that had coexistent corneal pathology such as pterygium and keratoconus or concurrent surgery such as trabeculectomy, corneal surgery or vitrectomy should be excluded. It is worth noting that the same sample of eyes containing the full range axial lengths can be used for optimizing the Hoffer Q, the Holladay 1 and the SRK/T even though the Hoffer Q formula is recommended for eyes shorter than 22 mm. If optimization relied only on eyes < 22 mm in length a very large number of cataract operations would be required to include 100 eyes under 22 mm in length.
With some electronic patient records or with optical biometers such as the Zeiss IOLMaster or the Haag-Streit Lenstar 900, there are inbuilt processes in its software for automatically calculating optimized IOL constants. When these are not available, data can be processed manually. One option is to use a validated iterative method where the IOL constant for each eye is retrospectively calculated so that the post operative prediction error is 0. These IOL constants are then averaged (after excluding 5% outliers on each side of the distribution). This method requires access to specialized software as well as having a high level of programming skills. A third option would be to manually estimate a close approximation of the optimized IOL constant, which does not require specialized IT skills or equipment. Using (1), (2), (3) and (4) from the list above, the predicted refractive error is calculated for each eye. Subtracting the post operative refraction from the predicted refraction in each eye gives the prediction error for each eye. When the average prediction error is calculated for a sample of 100 suitable eyes, the IOL constant is increased if the average prediction error is hyperopic or reduced if it is myopic. The magnitude of IOL constant alteration can be calculated in the following manner: For each 0.1 Dioptre of hyperopic error, the following adjustments need to be made: 0.06 for the pACD(Hoffer Q), 0.06 for the SF (Holladay 1) and 0.12 for the AC (SRK/T)[4]. For example, if for a specific IOL model the average prediction error for 100 appropriate eyes was +0.53D using a pACD of 4.97, an SF of 1.22 and an AC of 118.0, the following optimization would need to be made. For the pACD the new constant would be increased by 0.318 to 5.288, for the SF the constant would be increased by 0.318 to 1.538 and for the AC it would be increased by 0.636 to 118.636. In practice, the IOL constants for 3rd generation formulae are used to an accuracy of 2 decimal places for the pACD and the SF and one decimal place for the AC.
If the IOL constants are optimized and appropriate IOL formulae are used, refractive outcomes can be further improved when operating on the 2nd eye of a patient. It has been calculated[26-28] and prospectively validated[29] that the second eye can obtain improved refractive outcomes by modifying its refractive aim by half of the prediction error of the first eye. For example, if the first eye of a patient had a prediction error of +0.64D following surgery, the second eye should be aimed at -0.32D from the intended refraction. The requirements for this method to be applicable are: (1) in the bag IOL placement for both eyes; (2) the same IOL model and formula used in both eyes ;(3) interocular difference in keratometry of less than 0.6D; and (4) prediction error for the first eye +/-1.5D or less[27]. The same IOL formula should be used in both eyes and an optimized IOL constant should be used. This relationship has been demonstrated with the Hoffer Q, the Holladay 1, the SRK/T and the Olsen formulae. All papers were based on data obtained using optical biometry so this should not be used for eyes measured with contact ultrasound. No recommendation can be made for immersion ultrasound.
This article is aimed at a worldwide audience and aims to review the evidence on predicting spherical prediction error following cataract surgery, which globally is the most important refractive determinant of visual function following cataract surgery. In any surgical setting, may this be a tertiary centre in an economically fully developed country or an eye camp in a developing country, minimizing spherical refractive error should be one of the primary aims of cataract surgery. The relative importance of other factors that may be considered greatly depend on the resources available. These include the management of corneal astigmatism[30], concurrent correction for presbyopia using a variety of intraocular lens designs[31] and the use of femtosecond lasers[32]. It is not in the scope of this review to assess the evidence on the management in these situations.
Using an appropriate 3rd generation IOL formula with optimized constants and optical biometry with phacoemulsification and intracapsular IOL placement, around 70% and 95% of eyes can achieve refraction within ± 0.50D and ± 1.00D of the refractive target, respectively[14]. Using immersion ultrasound, similar results to optical biometry can be obtained as long as optimized IOL constants and appropriate 3rd generation IOL power formulae are used[33].
Refractive outcomes are a very important aspect of cataract surgery in any clinical setting. Good results primarily depend on the use of optimized IOL constants and appropriate IOL formulae. Clinical audit of post operative refraction is required in order to validate and refine IOL constants. The availability of optical biometry has enabled further improvements by the efficient capture of precise data but in departments where optical biometry is not available, very good outcomes can be obtained using modifications of immersion ultrasound technique and optimizing the IOL constants for the particular department.
P- Reviewer: Mimura T, Navas A, Wong J S- Editor: Song XX L- Editor: A E- Editor: Wu HL
| 1. | Briesen S, Roberts H, Lewallen S. The importance of biometry to cataract outcomes in a surgical unit in Africa. Ophthalmic Epidemiol. 2010;17:196-202. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 4] [Cited by in RCA: 6] [Article Influence: 0.4] [Reference Citation Analysis (0)] |
| 2. | George R, Rupauliha P, Sripriya AV, Rajesh PS, Vahan PV, Praveen S. Comparison of endothelial cell loss and surgically induced astigmatism following conventional extracapsular cataract surgery, manual small-incision surgery and phacoemulsification. Ophthalmic Epidemiol. 2005;12:293-297. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 52] [Cited by in RCA: 44] [Article Influence: 2.2] [Reference Citation Analysis (0)] |
| 3. | Bayramlar H, Hepsen IF, Yilmaz H. Myopic shift from the predicted refraction after sulcus fixation of PMMA posterior chamber intraocular lenses. Can J Ophthalmol. 2006;41:78-82. [RCA] [DOI] [Full Text] [Cited by in Crossref: 13] [Cited by in RCA: 15] [Article Influence: 0.8] [Reference Citation Analysis (0)] |
| 4. | Aristodemou P, Knox Cartwright NE, Sparrow JM, Johnston RL. Intraocular lens formula constant optimization and partial coherence interferometry biometry: Refractive outcomes in 8108 eyes after cataract surgery. J Cataract Refract Surg. 2011;37:50-62. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 86] [Cited by in RCA: 104] [Article Influence: 7.4] [Reference Citation Analysis (0)] |
| 5. | Ruit S, Tabin G, Chang D, Bajracharya L, Kline DC, Richheimer W, Shrestha M, Paudyal G. A prospective randomized clinical trial of phacoemulsification vs manual sutureless small-incision extracapsular cataract surgery in Nepal. Am J Ophthalmol. 2007;143:32-38. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 146] [Cited by in RCA: 133] [Article Influence: 7.4] [Reference Citation Analysis (0)] |
| 6. | Gogate PM. Small incision cataract surgery: Complications and mini-review. Indian J Ophthalmol. 2009;57:45-49. [PubMed] |
| 7. | Mallik VK, Kumar S, Kamboj R, Jain C, Jain K, Kumar S. Comparison of astigmatism following manual small incision cataract surgery: superior versus temporal approach. Nepal J Ophthalmol. 2012;4:54-58. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 15] [Cited by in RCA: 20] [Article Influence: 1.7] [Reference Citation Analysis (0)] |
| 8. | Hoffer KJ, Shammas HJ, Savini G. Comparison of 2 laser instruments for measuring axial length. J Cataract Refract Surg. 2010;36:644-648. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 87] [Cited by in RCA: 99] [Article Influence: 6.6] [Reference Citation Analysis (0)] |
| 9. | Shammas HJ. A comparison of immersion and contact techniques for axial length measurement. J Am Intraocul Implant Soc. 1984;10:444-447. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 74] [Cited by in RCA: 62] [Article Influence: 1.5] [Reference Citation Analysis (0)] |
| 10. | Findl O, Kriechbaum K, Sacu S, Kiss B, Polak K, Nepp J, Schild G, Rainer G, Maca S, Petternel V. Influence of operator experience on the performance of ultrasound biometry compared to optical biometry before cataract surgery. J Cataract Refract Surg. 2003;29:1950-1955. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 86] [Cited by in RCA: 89] [Article Influence: 4.0] [Reference Citation Analysis (0)] |
| 11. | Haigis W, Lege B, Miller N, Schneider B. Comparison of immersion ultrasound biometry and partial coherence interferometry for intraocular lens calculation according to Haigis. Graefes Arch Clin Exp Ophthalmol. 2000;238:765-773. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 471] [Cited by in RCA: 553] [Article Influence: 22.1] [Reference Citation Analysis (0)] |
| 12. | Durbin PLC. IAPB Standard List of Equipment, Drugs and Consumables for VISION 2020 Eye Care Service Units 2010/2011 [Internet]. Available from: http://www.lcif.org/EN/_files/pdfs/lcif_equipmentlist.pdf. |
| 13. | Durbin PLC; IAPB. IAPB Standard List [Internet] (2014). Available from: http://iapb.standardlist.org/. |
| 14. | Aristodemou P, Knox Cartwright NE, Sparrow JM, Johnston RL. Formula choice: Hoffer Q, Holladay 1, or SRK/T and refractive outcomes in 8108 eyes after cataract surgery with biometry by partial coherence interferometry. J Cataract Refract Surg. 2011;37:63-71. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 205] [Cited by in RCA: 237] [Article Influence: 16.9] [Reference Citation Analysis (0)] |
| 15. | Sheard RM, Smith GT, Cooke DL. Improving the prediction accuracy of the SRK/T formula: the T2 formula. J Cataract Refract Surg. 2010;36:1829-1834. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 54] [Cited by in RCA: 70] [Article Influence: 4.7] [Reference Citation Analysis (0)] |
| 16. | Haigis W. Occurrence of erroneous anterior chamber depth in the SRK/T formula. J Cataract Refract Surg. 1993;19:442-446. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 25] [Cited by in RCA: 27] [Article Influence: 0.8] [Reference Citation Analysis (0)] |
| 17. | Olsen T, Corydon L, Gimbel H. Intraocular lens power calculation with an improved anterior chamber depth prediction algorithm. J Cataract Refract Surg. 1995;21:313-319. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 118] [Cited by in RCA: 115] [Article Influence: 3.8] [Reference Citation Analysis (0)] |
| 18. | Olsen T. The C-constant: new concept in IOL power calculation and comparison with standard formulas (ESCRS Freepaper session 2012). Available from: http://www.escrs.org/milan2012/programme/free-paper-details.asp?id=13828&day=0. |
| 19. | Petermeier K, Gekeler F, Messias A, Spitzer MS, Haigis W, Szurman P. Intraocular lens power calculation and optimized constants for highly myopic eyes. J Cataract Refract Surg. 2009;35:1575-1581. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 69] [Cited by in RCA: 69] [Article Influence: 4.3] [Reference Citation Analysis (0)] |
| 20. | Sherwin JC, Dean WH, Schaefers I, Courtright P, Metcalfe N. Outcomes of manual small-incision cataract surgery using standard 22 dioptre intraocular lenses at Nkhoma Eye Hospital, Malawi. Int Ophthalmol. 2012;32:341-347. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 8] [Cited by in RCA: 8] [Article Influence: 0.6] [Reference Citation Analysis (0)] |
| 21. | Briesen S, Ng EY, Roberts H. Validity of first post-operative day automated refraction following dense cataract extraction. Clin Exp Optom. 2011;94:187-192. [RCA] [PubMed] [DOI] [Full Text] [Cited by in RCA: 1] [Reference Citation Analysis (0)] |
| 22. | Rabiu MM, Kyari F, Ezelum C, Elhassan E, Sanda S, Murthy GV, Sivasubramaniam S, Glibert C, Abdull MM, Abiose A. Review of the publications of the Nigeria national blindness survey: methodology, prevalence, causes of blindness and visual impairment and outcome of cataract surgery. Ann Afr Med. 2012;11:125-130. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 23] [Cited by in RCA: 27] [Article Influence: 2.1] [Reference Citation Analysis (0)] |
| 23. | Available from: http://www.augenklinik.uni-wuerzburg.de/ulib/. |
| 24. | Haigis W, Duzanec Z, Kammann J, Grehn F. Benefits of using three constants in IOL calculation (Poster at: Joint meeting, American Academy of Ophthalmology, Pan-American Association of Ophthalmology, October 24, 1999. Orlando FL). Available from: http://aao.scientificposters.com/epsSearchAAO.cfm, Search term: PO188. |
| 25. | Madge SN, Khong CH, Lamont M, Bansal A, Antcliff RJ. Optimization of biometry for intraocular lens implantation using the Zeiss IOLMaster. Acta Ophthalmol Scand. 2005;83:436-438. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 19] [Cited by in RCA: 19] [Article Influence: 1.0] [Reference Citation Analysis (0)] |
| 26. | Covert DJ, Henry CR, Koenig SB. Intraocular lens power selection in the second eye of patients undergoing bilateral, sequential cataract extraction. Ophthalmology. 2010;117:49-54. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 53] [Cited by in RCA: 53] [Article Influence: 3.3] [Reference Citation Analysis (0)] |
| 27. | Aristodemou P, Knox Cartwright NE, Sparrow JM, Johnston RL. First eye prediction error improves second eye refractive outcome results in 2129 patients after bilateral sequential cataract surgery. Ophthalmology. 2011;118:1701-1709. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 41] [Cited by in RCA: 45] [Article Influence: 3.2] [Reference Citation Analysis (0)] |
| 28. | Olsen T. Use of fellow eye data in the calculation of intraocular lens power for the second eye. Ophthalmology. 2011;118:1710-1715. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 57] [Cited by in RCA: 62] [Article Influence: 4.4] [Reference Citation Analysis (0)] |
| 29. | Jivrajka RV, Shammas MC, Shammas HJ. Improving the second-eye refractive error in patients undergoing bilateral sequential cataract surgery. Ophthalmology. 2012;119:1097-1101. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 38] [Cited by in RCA: 41] [Article Influence: 3.2] [Reference Citation Analysis (0)] |
| 30. | Maedel S, Hirnschall N, Chen YA, Findl O. Rotational performance and corneal astigmatism correction during cataract surgery: aspheric toric intraocular lens versus aspheric nontoric intraocular lens with opposite clear corneal incision. J Cataract Refract Surg. 2014;40:1355-1362. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 26] [Cited by in RCA: 33] [Article Influence: 3.0] [Reference Citation Analysis (0)] |
| 31. | Pepose JS, Qazi MA, Chu R, Stahl J. A prospective randomized clinical evaluation of 3 presbyopia-correcting intraocular lenses after cataract extraction. Am J Ophthalmol. 2014;158:436-446.e1. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 22] [Cited by in RCA: 25] [Article Influence: 2.3] [Reference Citation Analysis (0)] |
| 32. | Roberts TV, Lawless M, Bali SJ, Hodge C, Sutton G. Surgical outcomes and safety of femtosecond laser cataract surgery: a prospective study of 1500 consecutive cases. Ophthalmology. 2013;120:227-233. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 158] [Cited by in RCA: 179] [Article Influence: 13.8] [Reference Citation Analysis (0)] |
| 33. | Nemeth G, Nagy A, Berta A, Modis L. Comparison of intraocular lens power prediction using immersion ultrasound and optical biometry with and without formula optimization. Graefes Arch Clin Exp Ophthalmol. 2012;250:1321-1325. [RCA] [PubMed] [DOI] [Full Text] [Cited by in Crossref: 17] [Cited by in RCA: 23] [Article Influence: 1.8] [Reference Citation Analysis (0)] |